-
Dec 9, 2015
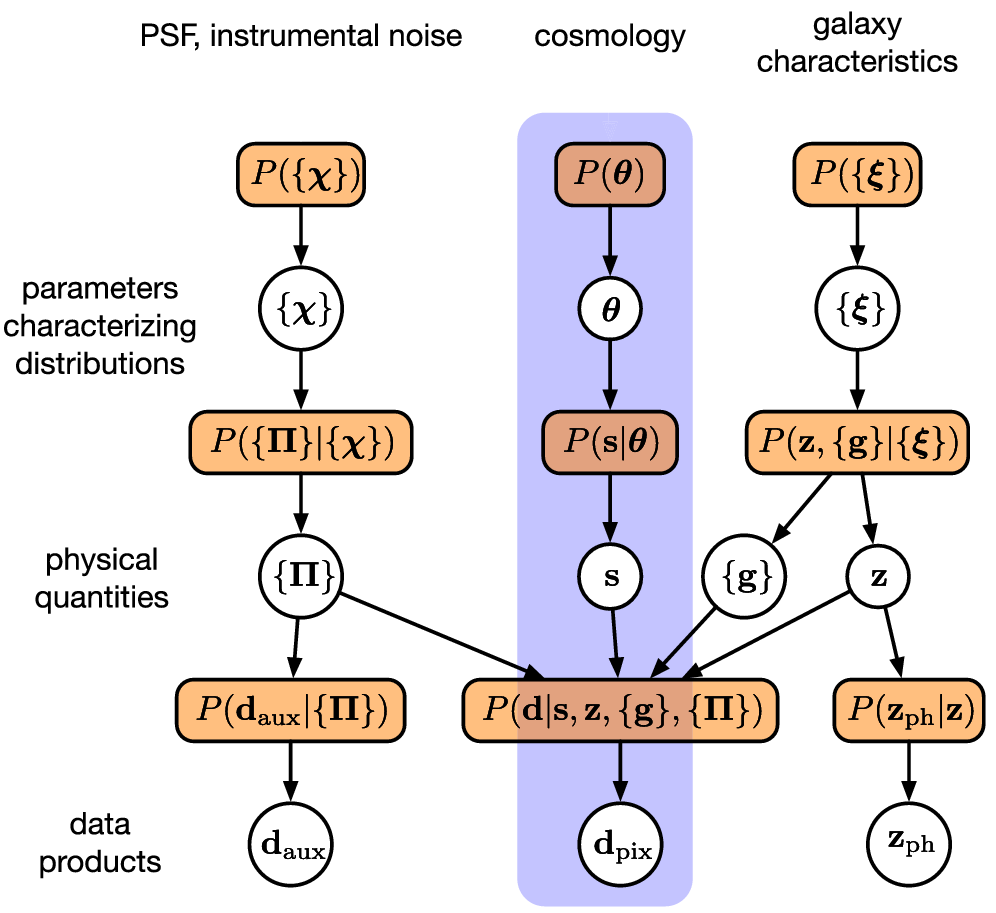We develop a Bayesian hierarchical modelling approach for cosmic shear power spectrum inference, jointly sampling from the posterior distribution of the cosmic shear field and its (tomographic) power spectra. Inference of the shear power spectrum is a powerful intermediate product for a cosmic shear analysis, since it requires very few model assumptions and can be used to perform inference on a wide range of cosmological models a posteriori without loss of information. We show that joint posterior for the shear map and power spectrum can be sampled effectively by Gibbs sampling, iteratively drawing samples from the map and power spectrum, each conditional on the other. This approach neatly circumvents difficulties associated with complicated survey geometry and masks that plague frequentist power spectrum estimators, since the power spectrum inference provides prior information about the field in masked regions at every sampling step. We demonstrate this approach for inference of tomographic shear E-mode, B-mode and EB-cross power spectra from a simulated galaxy shear catalogue with a number of important features; galaxies distributed on the sky and in redshift with photometric redshift uncertainties, realistic random ellipticity noise for every galaxy and a complicated survey mask. The obtained posterior distributions for the tomographic power spectrum coefficients recover the underlying simulated power spectra for both E- and B-modes.
-
Aug 27, 2013
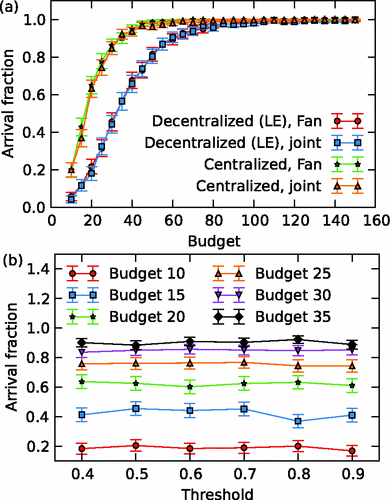We investigate algorithms to find short paths in spatial networks with stochastic edge weights. Our formulation of the problem of finding short paths differs from traditional formulations because we specifically do not make two of the usual simplifying assumptions: (1) we allow edge weights to be stochastic rather than deterministic and (2) we do not assume that global knowledge of a network is available. We develop a decentralized routing algorithm that provides en route guidance for travelers on a spatial network with stochastic edge weights without the need to rely on global knowledge about the network. To guide a traveler, our algorithm uses an estimation function that evaluates cumulative arrival probability distributions based on distances between pairs of nodes. The estimation function carries a notion of proximity between nodes and thereby enables routing without global knowledge. In testing our decentralized algorithm, we define a criterion that makes it possible to discriminate among arrival probability distributions, and we test our algorithm and this criterion using both synthetic and real networks.
-
Oct 8, 2012
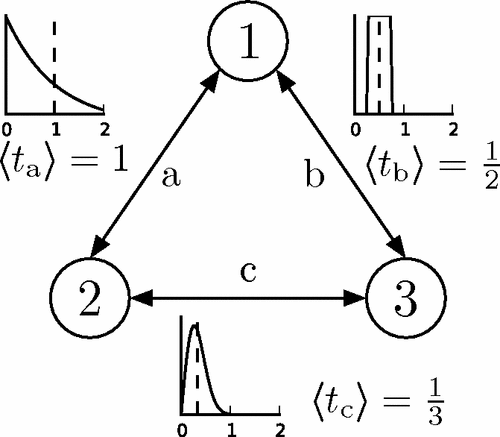The traditional way of studying temporal networks is to aggregate the dynamics of the edges to create a static weighted network. This implicitly assumes that the edges are governed by Poisson processes, which is not typically the case in empirical temporal networks. Accordingly, we examine the effects of non-Poisson inter-event statistics on the dynamics of edges, and we apply the concept of a generalized master equation to the study of continuous-time random walks on networks. We show that this equation reduces to the standard rate equations when the underlying process is Poissonian and that its stationary solution is determined by an effective transition matrix whose leading eigenvector is easy to calculate. We conduct numerical simulations and also derive analytical results for the stationary solution under the assumption that all edges have the same waiting-time distribution. We discuss the implications of our work for dynamical processes on temporal networks and for the construction of network diagnostics that take into account their nontrivial stochastic nature.